Research Focus
Composite Material and Sea Ice
I am interested in mathematical models that can be used to describe climate phenomena that involve advection enhanced difufsion processes, phase separation and solidification. Using analysis of the heat equation, modification of the Stefan problem and Stieltjes integrals I was able to obtain analytic bounds on the thermal conductivity in the presence of fluid flow, analytic bounds on the trapping constant and a coupled system describing the evolution of the marginal ice zone involving the ice concentration and heat diffusion.September 2017, invited speaker, Multi-scale modelling of ice characteristics and behaviour, Isaac Newton Institute for Mathematical Sciences, Cambridge, UK. See lecture here
Functionalized Cahn-Hilliard
The FCH is a higher-order free energy for blends of amphiphilic
polymers and solvent which balances solvation energy of ionic groups
against elastic energy of the underlying polymer backbone. Its gradient
flows describe the formation of solvent network structures which are
essential to ionic conduction in polymer membranes. The FCH possesses
stable, coexisting network morphologies and we characterize their
geometric evolution, bifurcation and competition through a center-stable
manifold reduction which encompasses a broad class of coexisting network
morphologies. The stability of the different networks is characterized by
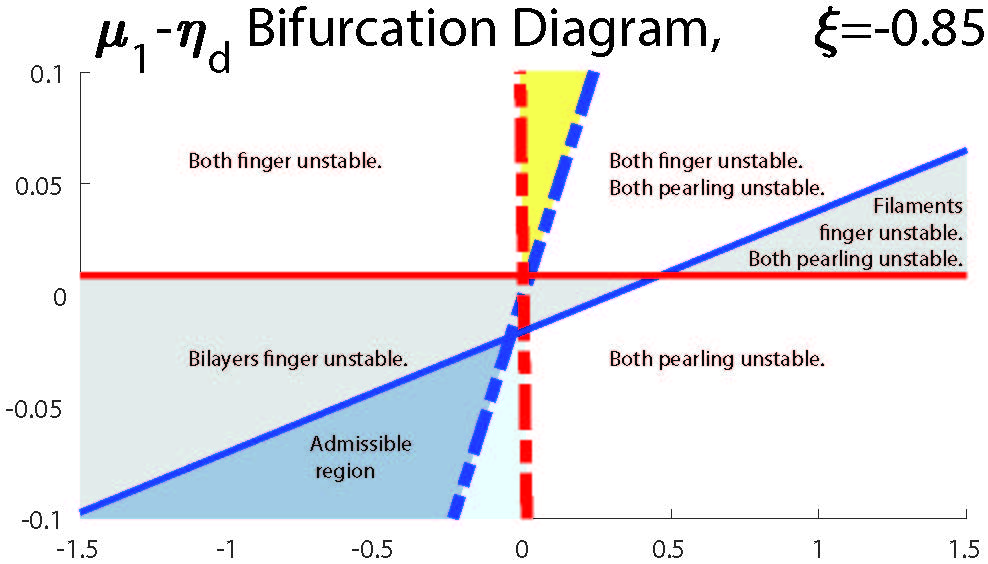
the meandering and pearling modes associated to the linearized system.
For the \(H^{-1}\) gradient flow of the FCH energy, using functional analysis and
asymptotic methods, we drive a sharp-interface geometric motion which
couples the flow of co-dimension 1 and 2 network morphologies, through the
far-field chemical potential. In particular, we derive expressions for the
pearling and meander eigenvalues for a class of far-from-self-intersection
co-dimension 1 and 2 networks, and show that the linearization is
uniformly elliptic off of the associated center stable space.
May 2015, mini-symposium organizer, SIAM: Conference on Applications of Dynamical Systems, Snowbird, UT. See lecture here
Publications
- N. Kraitzman & K. Promislow, (2014) An Overview of Network Bifurcations in the Functionalized Cahn-Hilliard Free Energy, editors: Jean Pierre Bourguignon, Rolf Jeltsch, Alberto Pinto, and Marcelo Viana, Mathematics of Energy and Climate Change: International Conference and Advanced School Planet Earth, Springer International Publishing, (pp. 191-214).
- N. Kraitzman & K. Promislow, (2018) Pearling Bifurcations in the Strong Functionalized Cahn-Hilliard Free Energy, SIAM Journal on Mathematical Analysis), Volume 50, Issue 3, pp.3395-3426. DOI
Under Review
- A. Christlieb, N. Kraitzman & K. Promislow, Competition and Complexity in Amphiphilic Polymer Morphology, Under review. arXiv/1711.00419
- N. Kraitzman, E. Cherkaev & K. Golden, Advection Enhanced Diffusion in a Porous Medium, Submitted.
Work in Progress
- N. Kraitzman, R. Hardenbrook, B. Murphy, E. Cherkaev, J. Zhu & K.Golden, Bounds on the Effective Thermal Conductivity of Sea Ice in the Presence of Fluid Convection, In preparation.
- N. Kraitzman, E. Cherkaev & K. Golden, Analytic Bounds on the Trapping Constant in Sea Ice, In preparation.
