


The slides for this talk are based on the assumption that the audience also attended the previous talk. That talk, however, does not enjoy the elaborate presence of this talk on the world wide web. So click here for a summary of
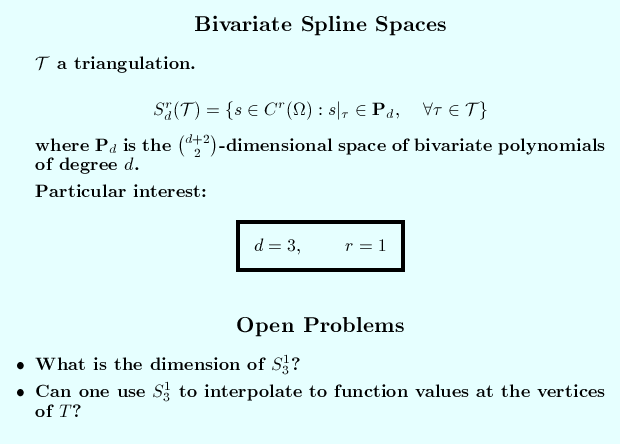
The space S13 is particularly interesting because it has the smallest possible polynomial degree for which the dimension is known to be larger than the number of vertices in the triangulation.
The question of the dimension of S13 is probably the most famous unsolved problem in this flavor of multivariate spline theory.
Of course, for any particular triangulation one can analyze an appropriate linear system and compute the dimension for that case. But we would like to have a formula that gives the dimension without having to solve a (large) linear system.
In general, everything gets easier as the polynomial degree increases. Dimension formulas for S1d are known if d > 3, and for Srd (where the spline is r times differentiable) exact dimension formulas are known when d > d3 +1, and the generic dimension is known when d = 3r+1.
When d> 3r+1 the smoothness conditions effectively decouple and the analysis can be done locally.
A special case of the spline spaces Srd are
triangle based finite elements.
[01-Nov-2023]