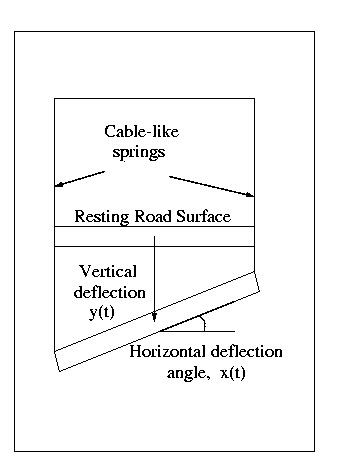
The model is based on the energy conservation principle that accounts for the kinetic and potential energies of a beam suspended by two "cable-like springs". And it accounts for the geometry shown in the figure to the right.

The model is based on the energy conservation principle that accounts
for the kinetic and potential energies of a beam suspended by two
"cable-like springs". And it accounts for the geometry shown in the
figure to the right.
McKenna's "cable-like springs" are very simple. The cables are assumed to be Hooke's law springs as long as the are stretched, but they do not resist compression. That is they are like a string, which resists stretching when taut, but doesn't resist compression. Mathematically, the force generated from such cable-like springs have the form
where k is the spring constant and d is the displacement from equilibrium of the spring.
The kinetic energy consists of two parts, the kinetic energy of vertical motion
and the kinetic energy of the torsional motion, which turns out to be
where L is the beam length.
Similarly the potential energy has two parts, -mgy the potential energy of the vertical displacement, and the potential energy due to torsional rotation angle x,
where the ( )+ means it contributes only if the term is positive, i.e. it accounts for the cable-like springs.
The equation of motion is derived by minimizing the total energy L = T - V, i.e. we differentiate the energy with respect to time and set the result equal to zero. Then a small viscous dissipation term is added and if the springs never lose tension, the resulting equation for the torsional motion is <
and for the vertical motion is